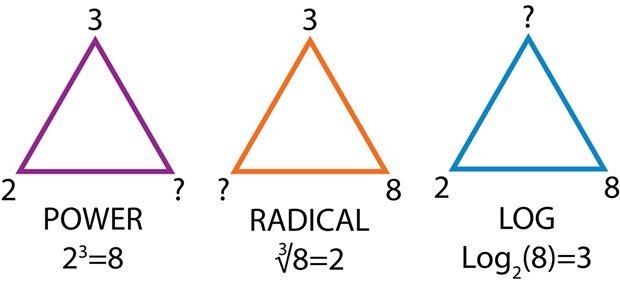The Log Connection 

"... an admirable artifice which, by reducing to a few days the labour of many months, doubles the life of the astronomer, and spares him the errors and disgust inseparable from long calculations."

— Pierre-Simon Laplace (1749-1827) on logarithms

At the start of the pandemic — seems so long ago! — the media showed us graphs of COVID's weekly progress. They were usually one of two types: "linear" or "logarithmic" (or "log"). Linear graphs had the number of cases on the vertical axis in even increments such as 0-1000-2000-3000 ... (adding 1,000 for each step), while logarithmic ones went 1-10-100-1,000-10,000 (multiplying by 10 for each step). Since the rise in the number of cases was roughly exponential (the more people infected, the faster the spread of the infection), the linear plot went up steeper and steeper with time, while the logarithmic plot approximated a straight line.

Which made sense if you understand logarithms, but I heard from plenty of folks who were put off by the awkward word and uncomfortable memories of school days. Blame Scottish polymath John Napier. He meant well but, in coming up with "logarithm" (from the Greek for ratio + number) early in the 17th century, he condemned future generations to fear and dread each time they encountered the word. Yet it's so much less complicated than it sounds.

The easiest way to understand logarithms, or logs, is with the power triangle (see illustration). Say you have three numbers, 2, 3 and 8, which you can connect by the equation 23 = 8 (2 x 2 x 2 = 8, or 2-cubed is 8). Another way of relating the three numbers is to write 3√ 8 = 2 (the cube root of 8 is 2). And yet another uses the log relationship: log2 (8) = 3 (log base 2 of 8 is 3), that is: "How many times do you have to multiply 2 by itself to get 8?" Note these express the same relationship between 2, 3 and 8 in different ways.

An example will make this clearer. Suppose a fast-growing tree doubles in height every year, starting at 1 foot. At the end of the first year, it's 2 feet high; at the end of the second year, it's 4 feet high; at the end of the third year, it's 8 feet, etc. If you encounter this tree when it's 64 feet high, how old is it? What you're really asking is, how many times did the tree's height have to double to get 64 feet? That is, 2-to-the-what-power equals 64? Using log notation, you're looking for the solution to log2 (64). (If you're feeling lazy, ask Siri or Alexa, "What's log base 2 of 64?") The answer is 6: The tree is 6 years old.

So, other than making pretty graphs, what's the big deal about logs? The answer lies in this beautiful relationship: log(ab) = log(a) + log(b). It doesn't look like much but without it, the advance of science would have been stymied between Napier's time and the advent of electronic calculators. Before Napier, multiplication had to be done the long way, the way we were taught in school. Remember plowing through, say, 3,578 x 874? But because log(ab) = log(a) + log(b), the log tables of my youth magically turned multiplication into addition (much easier and less error-prone). With a table of logs based on Napier's discovery, you could look up the logs of 3578 and 874, add them, then reverse the process (with antilog tables) to get the answer.

It's no exaggeration to say that log tables were essential to the scientific and industrial revolutions. And for engineering applications, where extreme precision wasn't needed, log-based slide rules enabled rapid calculation. Too bad this handy-dandy function is saddled with such a klunky word.

Barry Evans (he/him, [email protected]) had the same obsessive relationship with his slide rule that he now has with his iPhone.